Skip to content

Latest commit

 

History

History
401 lines (318 loc) · 15 KB

0040.组合总和II.md

File metadata and controls

401 lines (318 loc) · 15 KB
Raw

欢迎大家参与本项目，贡献其他语言版本的代码，拥抱开源，让更多学习算法的小伙伴们收益！

这篇可以说是全网把组合问题如何去重，讲的最清晰的了！

40.组合总和II

题目链接：https://leetcode-cn.com/problems/combination-sum-ii/

给定一个数组 candidates 和一个目标数 target ，找出 candidates 中所有可以使数字和为 target 的组合。

candidates 中的每个数字在每个组合中只能使用一次。

说明： 所有数字（包括目标数）都是正整数。 解集不能包含重复的组合。 

示例 1: 输入: candidates = [10,1,2,7,6,1,5], target = 8, 所求解集为: [ [1, 7], [1, 2, 5], [2, 6], [1, 1, 6] ]

示例 2: 输入: candidates = [2,5,2,1,2], target = 5, 所求解集为: [   [1,2,2],   [5] ]

思路

如果对回溯算法基础还不了解的话，我还特意录制了一期视频：带你学透回溯算法（理论篇） 可以结合题解和视频一起看，希望对大家理解回溯算法有所帮助。

这道题目和39.组合总和如下区别：

  1. 本题candidates 中的每个数字在每个组合中只能使用一次。
  2. 本题数组candidates的元素是有重复的，而39.组合总和是无重复元素的数组candidates

最后本题和39.组合总和要求一样，解集不能包含重复的组合。

本题的难点在于区别2中：集合（数组candidates）有重复元素，但还不能有重复的组合。

一些同学可能想了：我把所有组合求出来，再用set或者map去重，这么做很容易超时！

所以要在搜索的过程中就去掉重复组合。

很多同学在去重的问题上想不明白，其实很多题解也没有讲清楚，反正代码是能过的，感觉是那么回事，稀里糊涂的先把题目过了。

这个去重为什么很难理解呢，所谓去重，其实就是使用过的元素不能重复选取。 这么一说好像很简单！

都知道组合问题可以抽象为树形结构，那么“使用过”在这个树形结构上是有两个维度的，一个维度是同一树枝上使用过，一个维度是同一树层上使用过。没有理解这两个层面上的“使用过” 是造成大家没有彻底理解去重的根本原因。

那么问题来了，我们是要同一树层上使用过，还是统一树枝上使用过呢？

回看一下题目，元素在同一个组合内是可以重复的，怎么重复都没事，但两个组合不能相同。

所以我们要去重的是同一树层上的“使用过”，同一树枝上的都是一个组合里的元素，不用去重。

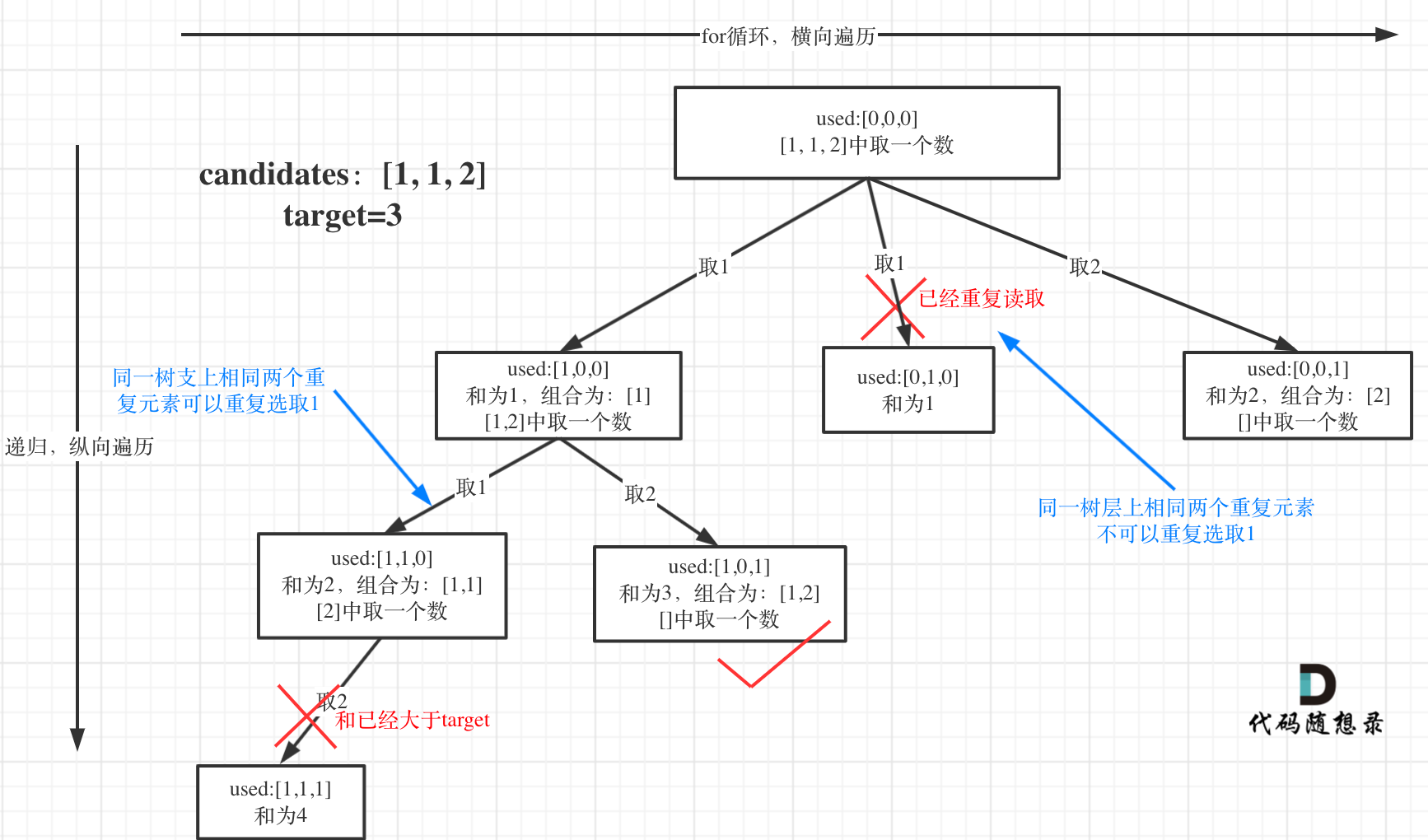
为了理解去重我们来举一个例子，candidates = [1, 1, 2], target = 3，（方便起见candidates已经排序了）

强调一下，树层去重的话，需要对数组排序！

选择过程树形结构如图所示：

40.组合总和II

可以看到图中，每个节点相对于 39.组合总和我多加了used数组，这个used数组下面会重点介绍。

回溯三部曲

  • 递归函数参数

与39.组合总和套路相同，此题还需要加一个bool型数组used，用来记录同一树枝上的元素是否使用过。

这个集合去重的重任就是used来完成的。

代码如下：

vector<vector<int>> result; // 存放组合集合
vector<int> path;           // 符合条件的组合
void backtracking(vector<int>& candidates, int target, int sum, int startIndex, vector<bool>& used) {
  • 递归终止条件

与39.组合总和相同，终止条件为 sum > target 和 sum == target。

代码如下：

if (sum > target) { // 这个条件其实可以省略
    return;
}
if (sum == target) {
    result.push_back(path);
    return;
}

sum > target 这个条件其实可以省略，因为和在递归单层遍历的时候，会有剪枝的操作，下面会介绍到。

  • 单层搜索的逻辑

这里与39.组合总和最大的不同就是要去重了。

前面我们提到：要去重的是“同一树层上的使用过”，如果判断同一树层上元素（相同的元素）是否使用过了呢。

如果candidates[i] == candidates[i - 1] 并且 used[i - 1] == false，就说明：前一个树枝，使用了candidates[i - 1]，也就是说同一树层使用过candidates[i - 1]。

此时for循环里就应该做continue的操作。

这块比较抽象，如图：

40.组合总和II1

我在图中将used的变化用橘黄色标注上，可以看出在candidates[i] == candidates[i - 1]相同的情况下：

  • used[i - 1] == true，说明同一树支candidates[i - 1]使用过
  • used[i - 1] == false，说明同一树层candidates[i - 1]使用过

这块去重的逻辑很抽象，网上搜的题解基本没有能讲清楚的，如果大家之前思考过这个问题或者刷过这道题目，看到这里一定会感觉通透了很多！

那么单层搜索的逻辑代码如下：

for (int i = startIndex; i < candidates.size() && sum + candidates[i] <= target; i++) {
    // used[i - 1] == true，说明同一树支candidates[i - 1]使用过
    // used[i - 1] == false，说明同一树层candidates[i - 1]使用过
    // 要对同一树层使用过的元素进行跳过
    if (i > 0 && candidates[i] == candidates[i - 1] && used[i - 1] == false) {
        continue;
    }
    sum += candidates[i];
    path.push_back(candidates[i]);
    used[i] = true;
    backtracking(candidates, target, sum, i + 1, used); // 和39.组合总和的区别1：这里是i+1，每个数字在每个组合中只能使用一次
    used[i] = false;
    sum -= candidates[i];
    path.pop_back();
}

注意sum + candidates[i] <= target为剪枝操作，在39.组合总和有讲解过！

C++代码

回溯三部曲分析完了，整体C++代码如下：

class Solution {
private:
    vector<vector<int>> result;
    vector<int> path;
    void backtracking(vector<int>& candidates, int target, int sum, int startIndex, vector<bool>& used) {
        if (sum == target) {
            result.push_back(path);
            return;
        }
        for (int i = startIndex; i < candidates.size() && sum + candidates[i] <= target; i++) {
            // used[i - 1] == true，说明同一树支candidates[i - 1]使用过
            // used[i - 1] == false，说明同一树层candidates[i - 1]使用过
            // 要对同一树层使用过的元素进行跳过
            if (i > 0 && candidates[i] == candidates[i - 1] && used[i - 1] == false) {
                continue;
            }
            sum += candidates[i];
            path.push_back(candidates[i]);
            used[i] = true;
            backtracking(candidates, target, sum, i + 1, used); // 和39.组合总和的区别1，这里是i+1，每个数字在每个组合中只能使用一次
            used[i] = false;
            sum -= candidates[i];
            path.pop_back();
        }
    }

public:
    vector<vector<int>> combinationSum2(vector<int>& candidates, int target) {
        vector<bool> used(candidates.size(), false);
        path.clear();
        result.clear();
        // 首先把给candidates排序，让其相同的元素都挨在一起。
        sort(candidates.begin(), candidates.end());
        backtracking(candidates, target, 0, 0, used);
        return result;
    }
};

补充

这里直接用startIndex来去重也是可以的， 就不用used数组了。

class Solution {
private:
    vector<vector<int>> result;
    vector<int> path;
    void backtracking(vector<int>& candidates, int target, int sum, int startIndex) {
        if (sum == target) {
            result.push_back(path);
            return;
        }
        for (int i = startIndex; i < candidates.size() && sum + candidates[i] <= target; i++) {
            // 要对同一树层使用过的元素进行跳过
            if (i > startIndex && candidates[i] == candidates[i - 1]) {
                continue;
            }
            sum += candidates[i];
            path.push_back(candidates[i]);
            backtracking(candidates, target, sum, i + 1); // 和39.组合总和的区别1，这里是i+1，每个数字在每个组合中只能使用一次
            sum -= candidates[i];
            path.pop_back();
        }
    }

public:
    vector<vector<int>> combinationSum2(vector<int>& candidates, int target) {
        path.clear();
        result.clear();
        // 首先把给candidates排序，让其相同的元素都挨在一起。
        sort(candidates.begin(), candidates.end());
        backtracking(candidates, target, 0, 0);
        return result;
    }
};

总结

本题同样是求组合总和，但就是因为其数组candidates有重复元素，而要求不能有重复的组合，所以相对于39.组合总和难度提升了不少。

关键是去重的逻辑，代码很简单，网上一搜一大把，但几乎没有能把这块代码含义讲明白的，基本都是给出代码，然后说这就是去重了，究竟怎么个去重法也是模棱两可。

所以Carl有必要把去重的这块彻彻底底的给大家讲清楚，就连“树层去重”和“树枝去重”都是我自创的词汇，希望对大家理解有帮助！

其他语言版本

Java：

class Solution {
    List<List<Integer>> lists = new ArrayList<>();
    Deque<Integer> deque = new LinkedList<>();
    int sum = 0;

    public List<List<Integer>> combinationSum2(int[] candidates, int target) {
        //为了将重复的数字都放到一起，所以先进行排序
        Arrays.sort(candidates);
        //加标志数组，用来辅助判断同层节点是否已经遍历
        boolean[] flag = new boolean[candidates.length];
        backTracking(candidates, target, 0, flag);
        return lists;
    }

    public void backTracking(int[] arr, int target, int index, boolean[] flag) {
        if (sum == target) {
            lists.add(new ArrayList(deque));
            return;
        }
        for (int i = index; i < arr.length && arr[i] + sum <= target; i++) {
            //出现重复节点，同层的第一个节点已经被访问过，所以直接跳过
            if (i > 0 && arr[i] == arr[i - 1] && !flag[i - 1]) {
                continue;
            }
            flag[i] = true;
            sum += arr[i];
            deque.push(arr[i]);
            //每个节点仅能选择一次，所以从下一位开始
            backTracking(arr, target, i + 1, flag);
            int temp = deque.pop();
            flag[i] = false;
            sum -= temp;
        }
    }
}

Python：

class Solution:
    def combinationSum2(self, candidates: List[int], target: int) -> List[List[int]]:
        res = []
        path = []
        def backtrack(candidates,target,sum,startIndex):
            if sum == target: res.append(path[:])
            for i in range(startIndex,len(candidates)):  #要对同一树层使用过的元素进行跳过
                if sum + candidates[i] > target: return 
                if i > startIndex and candidates[i] == candidates[i-1]: continue  #直接用startIndex来去重,要对同一树层使用过的元素进行跳过
                sum += candidates[i]
                path.append(candidates[i])
                backtrack(candidates,target,sum,i+1)  #i+1:每个数字在每个组合中只能使用一次
                sum -= candidates[i]  #回溯
                path.pop()  #回溯
        candidates = sorted(candidates)  #首先把给candidates排序，让其相同的元素都挨在一起。
        backtrack(candidates,target,0,0)
        return res

Go：

主要在于如何在回溯中去重

func combinationSum2(candidates []int, target int) [][]int {
    var trcak []int
    var res [][]int
    var history map[int]bool
    history=make(map[int]bool)
    sort.Ints(candidates)
    backtracking(0,0,target,candidates,trcak,&res,history)
    return res
}
func backtracking(startIndex,sum,target int,candidates,trcak []int,res *[][]int,history map[int]bool){
    //终止条件
    if sum==target{
        tmp:=make([]int,len(trcak))
        copy(tmp,trcak)//拷贝
        *res=append(*res,tmp)//放入结果集
        return
    }
    if sum>target{return}
    //回溯
    // used[i - 1] == true，说明同一树支candidates[i - 1]使用过
    // used[i - 1] == false，说明同一树层candidates[i - 1]使用过
    for i:=startIndex;i<len(candidates);i++{
        if i>0&&candidates[i]==candidates[i-1]&&history[i-1]==false{
                continue
        }
        //更新路径集合和sum
        trcak=append(trcak,candidates[i])
        sum+=candidates[i]
        history[i]=true
        //递归
        backtracking(i+1,sum,target,candidates,trcak,res,history)
        //回溯
        trcak=trcak[:len(trcak)-1]
        sum-=candidates[i]
        history[i]=false
    }
}

javaScript：

/**
 * @param {number[]} candidates
 * @param {number} target
 * @return {number[][]}
 */
var combinationSum2 = function(candidates, target) {
    const res = []; path = [], len = candidates.length;
    candidates.sort();
    backtracking(0, 0);
    return res;
    function backtracking(sum, i) {
        if (sum > target) return;
        if (sum === target) {
            res.push(Array.from(path));
            return;
        }
        let f = -1;
        for(let j = i; j < len; j++) {
            const n = candidates[j];
            if(n > target - sum || n === f) continue;
            path.push(n);
            sum += n;
            f = n;
            backtracking(sum, j + 1);
            path.pop();
            sum -= n;
        }
    }
};